Simplify the polynomial (7m2-9n)-(6m3+7m2+5n) (Elementary algebra)
C is the best option. Solve the expression (7m2-9n)-(6m3+7m2+5n) =7m2-9n-6m3-7m2-5n = -6m3-14n A is incorrect because 6m3 should carry a negative sign. B is incorrect because the negative values have not been kept in mind while solving the polynomial. D is incorrect as signs have been reversed. E is incorrect as it does not reflect the final answer, we get after solving the expression.
If f(x)= x2-4 and g(x)=2x+1, then g(f(x))=? (intermediate algebra)
B is the best option. To find g(f(x)) we will substitute the value of f(x) into g(x) =2(x2-4) +1 =2x2-7 A is incorrect as it reflects the value of f(g(x)). C is incorrect because it is the expanded value for f(g(x)).. D and E are also incorrect as they do not reflect the value of g(f(x)).
Factor x2+7x+6 (elementary algebra)
C is the best option. To factor we will expand the polynomial as x2+6x+x+6 =(x+6) (x+1) A, B,D and E are incorrect as they have not been factored correctly and you can check that after multiplying both the factors in these incorrect option we will not get the original expression i.e. x2+7x+6.
Solve the absolute inequality given below. (Intermediate algebra) 14>|n|
C is the best option. The absolute value of n, includes all numbers between 14 and -14 (excluding 14 and -14). Thus, we will write it in the form of 14 > n > -14. A is not true because the signs of inequality used are incorrect. B cannot be true as it is the question of inequality. D and E are also incorrect because neither n= 0 nor n≥14.
x2.x-3 is equivalent to(Elementary algebra)
E is the best option. The negative exponent of x can be written as 1/x, therefore, x-3will be written as 1/x3. So, overall, we can write the expression as x2/x3. A is incorrect as it has simply employed the operation of addition between the two powers of x. B is incorrect as it has multiplied the power of x. C is incorrect because it has added the powers of x by ignoring the negative sign. D is also incorrect as the powers are multiplied despite the fact that one power of x is negative.
Suppose that a point F is located in the one of the four quadrants below. If the point F has non- zero x and y coordinates and both have same sign, then in which quadrant F is located? (Coordinate geometry)
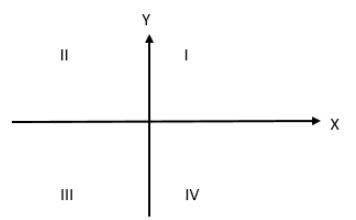
C is the best option. We can see that in quadrant I, both x and y have the same sign i.e. positive. Similarly, in quadrant III both the coordinates have the same negative sign. Therefore, both I and III fulfill the condition of the same sign. A cannot be true because besides I quadrant, quadrant III also contains the same sign x and y coordinates. B is also incorrect because of the same reason described for choice B. D and E cannot be true as quadrants II and IV have x and y coordinates with opposite signs.
Two points A and B are located in the x- y coordinate below. Find the distance between A and B. (Coordinate geometry)
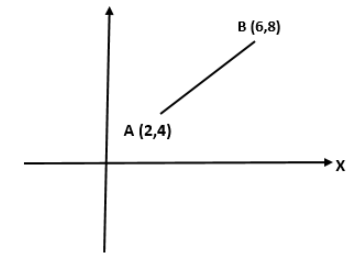
B is the best option. The formula for distance is √(x2-x1)2+(y2-y1)2 Putting the values in this formula, we get √(6-2)2+(8-4)2 = √16+16 =√32 =5.65 A , C , D and E are all incorrect because putting the values of x and y coordinates in the distance formula we do not get this resultant distance between the point A and B.
Two points are located in x and y coordinate plane. Find the midpoint of these two points. (coordinate geometry)
C is the best option. The coordinates for midpoint are calculated through formula =x1+x2/2 , y1+y2/2 = 4-4/2,3+1/2 =0,2 A is incorrect because it is the value of (y,x) of the midpoint. B is incorrect because it is calculated through a wrong formula x1-x2/2 , y1-y2/2 . D is incorrect because it is the value of (y, x) calculated through the formula used in the previous incorrect option. E is incorrect because putting values in the midpoint formula, we do not get these x and y coordinates for the midpoint.
What is slope of the line defined by the equation 7x+2y=36? (Coordinate geometry)
E is the best option. To find the slope, first convert the equation in slope intercept form y=mx+b 2y=-7x+36 y=-7⁄2 A is incorrect as it is the coefficient value of variable x. B is incorrect as it is the coefficient of y. C is incorrect because it does not reflect the value of slope m. D is not true because it is the value of b not m.
If sin θ=0.707 and tan θ=1.73, then cos θ =? (trigonometry)
B is the best option. We know that tan θ= sinθ/cosθ 1.73=0.707/cosθ Cos θ = 0.707/1.73 =0.408 A is not correct because we do not get 0.50 after putting values in the formula of tan θ and solving it. C is incorrect as tan θ and sin θ values are multiplied with each other which is incorrect. D is incorrect because we will solve the question by dividing sin θ by tan θ instead of tan θ by sin θ. E is incorrect as the values are subtracted with each other which cannot be correct.
If 4x-3y=13 and 7y-x=3, then x+y=? (Intermediate algebra)
D is the best option. 7y-x=3 7y-3=x Substitute this value in the first equation 4(7y-3)-3y=13 28y-12-3y=13 25y=25 y=1 Substitute the value of y in either equation 7(1)-x=3 7-x=3 X=4 x+y=1+4 =5 A is not true because it is the value of x-y. B is incorrect because it is the value of x only. C is incorrect because it is the value of y only. E is incorrect because we do not get this value for x+y.
If A=|3 4 -1 -5 | and B= |-2 3 -4 -2 | , then find A-B. (Intermediate algebra)
C is the best option. A-B=|3-(-2) 4-3 -1-(-4) -5-(-2) | =|3+2 1 -1+4 -5+2 | =|5 1 3 -3 | A is not true because it reflects A+B.B, D and E are incorrect because they do not reflect A-B.
The exponential form of logx243=3 is: (Intermediate algebra)
A is the best option. The exponential form of the equation x=abis logax=b. Therefore, we can write logx243=3 as x3=243. B, C,D and E are incorrect because they do not reflect the correct exponential form of logx243=3.
There are total t cups in the bucket after Alice added n more cups in it. Alice then took out n-5 cups from the bucket. How much water is left in the bucket? (Pre- algebra)
E is the best option. Total number of cups= t Cups that Alice took out=-n-5 Cups left in the bucket= t-(n-5) =t-n+5 A is not true as Alice took out n-5 cups not 5 cups from the bucket. B is incorrect as it reflects total number of cups before Alice took out water from the bucket. C is incorrect because Alice took out n-5 cups, not n cups from the bucket. D is incorrect because after subtracting n-5 from t we will get t-n+5 instead of t-n-5.
If you multiply the inequality 3x-7y≥14 by -3, the resultant inequality will be: (Elementary algebra)
C is the best option. We will simply multiply each term by -3 and reverse the inequality sign as when an inequality is multiplied by a negative number the sign is reversed. The resultant inequality will be-9x+21y≤-42. A is incorrect because the sign is not reversed. Similarly, B and D are also incorrect as multiplication is not done properly and signs are also the same. E is incorrect because when -7y is multiplied by -3 we get +21y instead of -21y.